In a previous article we discussed how to calculate the cylinder force. In this article we will take a look at the speed at which the cylinder moves in and out. This is dependent on two main factors: the flow of the medium and the volume the medium has to fill to displace the piston a certain amount. The time it takes to fully extend or retract the piston is determined by the speed that comes forth out of the latter two and the stroke length of the cylinder.
If you do not want the theoretical background and just go on with it, download our cylinder calculation sheet for free!
Theoretical
To put into formulas what is stated above:
General cylinder speed formula:
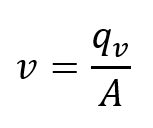
v = speed of the piston in (m/s) or (m/min) or (cm/s)
A = Surface area in (m2) or (mm2) or (cm2)
qv = Flow in (L/s) or (L/min)
Speed out (extend)
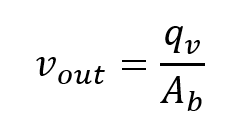
Speed in (retract)

With the calculated speed we can than easily calculate the time it takes to fully extend or retract the cylinder. Since the ring surface is always smaller than the bore, retracting is always faster then extending. The difference between the two is expressed by the ratio phi (unitless). With this factor we can quickly calculate the extend or retract speed desired when we know one or the other. With this info we can set out to determine the size of our cylinder (considering the force of the cylinder at a given size). The smaller the cylinder, the more speed. (At constant flow)
Stroke time:
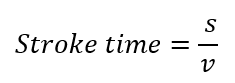
Stroke time = the time it takes to move the piston fully in or out in (s)
s = stroke of the cylinder in (m) or (cm)
v = speed of the piston in (m/s) or (m/min) or (cm/s)
Stroke time out:

Stroke time in:
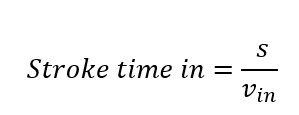
OR
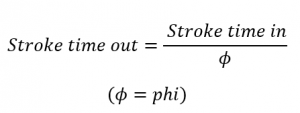
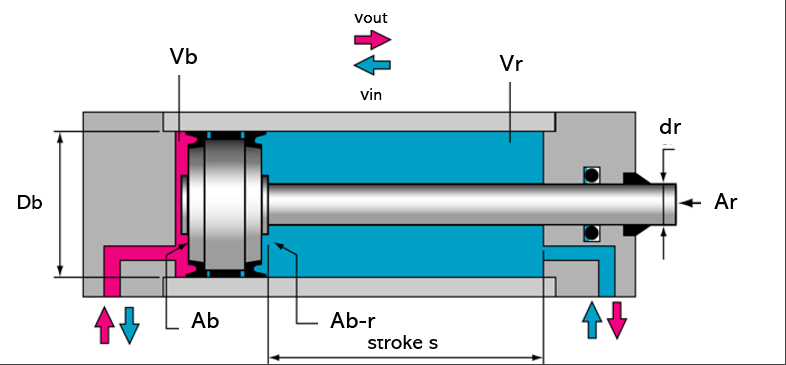
Example 1
Data:
Vout = 5 m/min = 50 dm/min
qv = 10 L/min = 10 dm3
Requested:
Ab = ?
Formula:
Ab = qv / vout
Solution:
Ab = 10 / 50 = 0,2 dm2 = 20 cm2
Example 2
Data:
vin = 16 m/min = 160 dm/min
Ab-r = 12 cm2 = 0,12 dm2
Requested:
qv = ?
Formula:
qv = vin * Ab-r
Solution:
qv = 160 * 0,12 = 19,2 L/min
TIP: always try to convert to dm because the normal flow unit is L/min. 1L = 1 dm3.

Add a comment